4G  Payout Annuities

A payout annuity works like a home mortgage, except that you turn the tables on the bank - you become the lender and the bank is the borrower. You give the bank a sum of money, and the bank pays you back with regular and equal payments over a specified time period. The bank pays simple interest on the outstanding balance, just as you would do with a home mortgage.

Payout annuities are popular with retired people who have saved some money over their working years, possibly in a retirement plan or through a savings annuity. Instead of taking possession of all their savings at once, they deposit them in a payout annuity earning interest, while taking out regular payments for living expenses.

The relevant formulas for a payout annuity are the same as those for a simple interest amortized loan; for convenience we repeat those formulas here. The notation is

The formula specifying the payment amount p is

Solving this formula for the principal P gives

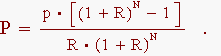
example 1

Mr. Harrison is ready to retire, having already bought his golf clubs. He has accumulated $300,000 in savings, and he will deposit this amount in a 20-year payout annuity with an annual interest rate of 6.6%. We calculate

1. his monthly payment to be received from the annuity,
2. the total amount he will receive from the annuity,
3. the total interest he will earn from the annuity.

The principal is P = $300,000, the annual interest rate is r = .066, and the periodic rate is R = r/12 = .066/12 = .0055. Mr. Harrison will make 12 payments a year for 20 years, for a total of N = 20 · 12 = 240 payments. The formula gives his monthly payment from the annuity as

Over 20 years, receiving 240 payments, Mr. Harrison will receive from the annuity the total amount

The amount of interest he will earn is the difference between the amount he takes out and his initial principal, or

example 2

Elizabeth is contemplating retirement in a few years. From the Bank of England she can get a 25-year payout annuity at a 6% annual interest rate. If she wants her monthly payments to be $5000, how much should she deposit into the annuity?

We use the formula giving the principal P in terms of the other variables. The annual interest rate is r = .06, and the periodic rate is R = r/12 = .06/12 = .005. The number of monthly payments over 25 years will be 12 · 25 = 300. As Elizabeth wants the monthly payment to be p = $5000, we find that her required principal is

example 3

A young newly married couple has just opened a 40-year savings annuity, making monthly payments of $100 at a 5.4% annual interest rate. Their plan is that, after expiration of the annuity in 40 years, they will deposit the balance into a 25-year payout annuity at the same interest rate. We calculate

1. the total amount they will pay into the savings annuity,
2. their amount in the savings annuity at expiration,
3. their monthly payment from the payout annuity,
4. the total amount they will receive from the payout annuity.

The number of payments the couple makes into the savings annuity will be 12 · 40 = 480, and so the amount they will pay into that annuity is

For each annuity the annual interest rate is r = 5.4% = .054, and the monthly rate is R = r/12 = .0045. In the savings annuity we have p = $100 and N = 480, and so the formula for the expiration amount A in the first annuity gives

The expiration amount in the first annuity becomes the principal in the second annuity. The number of payments in the second annuity is N = 12 · 25 = 300, and the monthly interest rate is R = .0045; thus the formula for the payment in the payout annuity gives the monthly payment

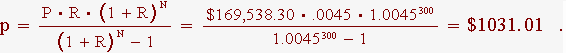
We multiply this payment amount by the number of payments 300, and find that the total amount that the couple will receive from the payout annuity is

Note that the difference in what the couple receives in the payout annuity and what they pay into the savings annuity is

This striking difference highlights the advantage of beginning a savings plan early in life.

EXERCISES 4G

1. For their retirement years Mr. and Mrs. Johnson have invested $120,000 into a 30-year payout annuity, paying interest at an annual rate of 6.3%. Calculate
   1. the size of each of their monthly payments,
   2. the total amount they will receive from the annuity,
   3. the total interest they will earn from the annuity.
   
   
2. Suppose the Johnsons wanted to receive each month exactly $1000 from their annuity. With the same 6.3% interest rate and the same 30-year term, what would their principal have to be in order to earn this monthly payment?
   
   
3. The Johnsons could alternatively invest their $120,000 into a 20-year payout annuity, paying interest at a 6% annual rate. What would be their monthly payment under this plan? What would be the total amount they receive from this annuity?

		When she was 16 years old Meina's grandfather passed away, leaving her $1,000,000 in a 50 year payout annuity paying interest at 5.1% a year. Calculate the amount of Meina's monthly payment, the total amount Meina will collect from the annuity, the total interest Meina will earn from the annuity.

5. The Nickersons are thinking of depositing $80,000 of their savings into a payout annuity. After asking around at various banks, they have narrowed their choices to three plans:

   	(A)  15-year annuity, 4.8% annual interest,
   	(B)  20-year annuity, 5.4% annual interest,
   	(C)  25-year annuity, 6% annual interest.

   Calculate their monthly payment under each plan, as well as the total amount they will collect from the annuity under each plan.
   
   
6. Ewalina, 18 years old, intends to deposit $200 each month into a 50-year savings annuity, paying interest at the rate of 4.8% per year. After expiration of this annuity, she will invest the balance into a 20 year payout annuity, paying interest at the same rate. Calculate
   1. the total amount she will pay into the savings annuity,
   2. the balance in the savings annuity at expiration in 50 years,
   3. her monthly payment in the payout annuity,
   4. the total amount she will collect from the payout annuity.

Mr. Chen, a 30-year-old chef at a Waikiki hotel, figures he will be around about 50 more years. He wants to spend his next 30 years making monthly payments into a savings annuity, paying interest at 6% per year. He intends then to turnover his balance into a 20-year payout annuity at the same interest rate, from which he will collect monthly payments until he is 80. If Mr. Chen wants payments of $3000 per month from the payout annuity, what then should be the size of his payments into the savings annuity?