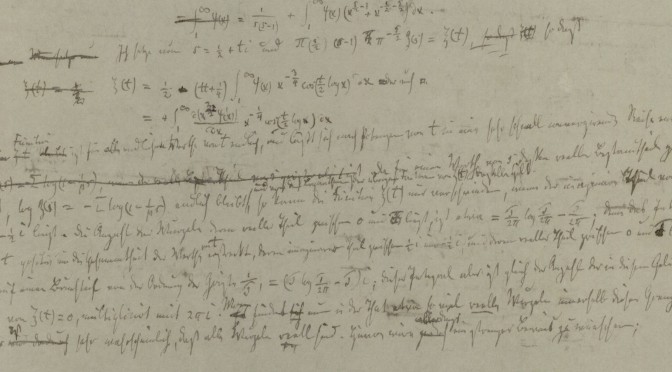UNDERGRADUATE MATH MAJORS ADVISING FOR FALL 2024 REGISTRATION
WHEN: Monday, April 7, 2024
TIME: 5:00pm – 7:00pm
WHERE: Keller Hall Room 302
RSVP Required: Check your @hawaii.edu email for the RSVP Form
Learn about MATH courses offered in Fall 2024, as well as career opportunities, possible internships, the MATH Club, and research fields that majoring in MATH has to offer.
Speakers
From UH Manoa’s Department of Mathematics unless otherwise indicated.
- 5:00 Bjørn Kjos-Hanssen, Moderator and Associate Chair
Welcome - 5:05 Mirjana Jovovic, Director of Undergraduate Studies
Fall 2024 Class Availability in Math;
Tutor/Grader program; Benson Farb’s talks - 5:10 Yash Lodha, Assistant Professor: Math 421, Topology
- 5:15 Ellen Hughes and Jasmine Carpena, undergraduate students and Math Club co-presidents
Math Club Slides - 5:20 Chuang Xu, Assistant Professor: Math 471, Probability. Advising_for_471
- 5:25 Farzana Nasrin, Assistant Professor: Careers in Mathematics. Careers in Math; Math 407 (Numerical Analysis) syllabus_407_Fall_2024 (1)
- 5:30 Pavel Guerzhoy, Professor: Math 412, Abstract Algebra; Putnam Exam.
- 5:35 George Wilkens, Professor: Professor Guentner’s Math 353 class.
- 5:40 (maybe) Asaf Hadari, Associate Professor & Graduate Chair: Math 442, Vector Analysis
- 5:45 Pizza, and get your forms signed and discuss courses for Spring with Willett, Kjos-Hanssen, Jovovic, etc.
See also: BAP program, Program sheets
Information about the 2023 event
UNDERGRADUATE MATH MAJORS ADVISING FOR SPRING 2024 REGISTRATION
WHEN: Wednesday, November 1, 2023
TIME: 5:00pm – 7:00pm
WHERE: Keller Hall Room 303
RSVP Required: Check your @hawaii.edu email for the RSVP Form
Learn about MATH courses offered in Spring 2024, as well as career opportunities, possible internships, the MATH Club, and research fields that majoring in MATH has to offer.
Speakers
From UH Manoa’s Department of Mathematics unless otherwise indicated. Speaker n will yield the floor to Speaker n+1 by the time indicated in Speaker n+1′s line.
- 5:00 Bjørn Kjos-Hanssen, Moderator and Associate Chair
Welcome - 5:01 Mirjana Jovovic, Director of Undergraduate Studies
Spring 2024 Class Availability in Math - 5:08 Farzana Nasrin, Assistant Professor (with a surprise appearance by Prof. Wilkens)
Data science track - 5:15 Seanyelle Yagi, Associate Specialist in Mathematics Education (with a surprise appearance by Ka’eo Lee)
Mathematics teaching opportunities - 5:22 Asaf Hadari, Associate Professor & Graduate Chair
Graduate Programs - 5:29 Yash Lodha, Assistant Professor; Ellen Hughes and Jasmine Carpena, undergraduate students and Math Club co-presidents
Math Club - 5:36 April Legaspi and Jordan Abanto, College of Natural Sciences Student Academic Success Center Advising
Ambassador Program, Gen Ed requirements. - 5:43 Rico Vicente, Graduate Assistant in Ph.D. Program
Research Experiences for Undergraduates (REUs) - 5:50 John Robertson, Instructor
Learning Assistant Program, Learning Emporium - 5:57 Pizza, and get your forms signed and discuss courses for Spring with Willett, Kjos-Hanssen, Dovermann, Jovovic, etc.
See also: BAP program, Program sheets
Information about the 2022 event
Group advising for Spring 2023 takes place November 2, 2022.
KELLER HALL ROOM 303 from 5 pm
- 6:30 pm
Speakers and events
| Time | Speaker | Title |
|---|---|---|
| 5:00pm | Bjørn Kjos-Hanssen | Welcome, and Actuarial science program |
| 5:02pm | Mirjana Jovovic | Spring 2023 math courses |
| 5:05pm | Michael Joyce | A Meandering Path Towards a Career in Data Science – One Math Grad’s Journey |
| 5:18pm | Yuriy Mileyko | Data science track |
| 5:25pm | Sean Sanford | Pure math and academia |
| 5:38pm | Evan Gawlik | Computational science track and tutor program |
| 5:45pm | Rufus Willett | Practical Learning Assistant things |
| 5:50pm | Solimar Carrasquillo Ho | Student academic success center |
| 6:00pm | Break-out Sessions & Pizza |
More info about being a Learning Assistant
Organizer: Alicia Maedo